Our curiosity is getting the best of us. We need to see the parabola's new parts in action. Let's check out one of their graphs:
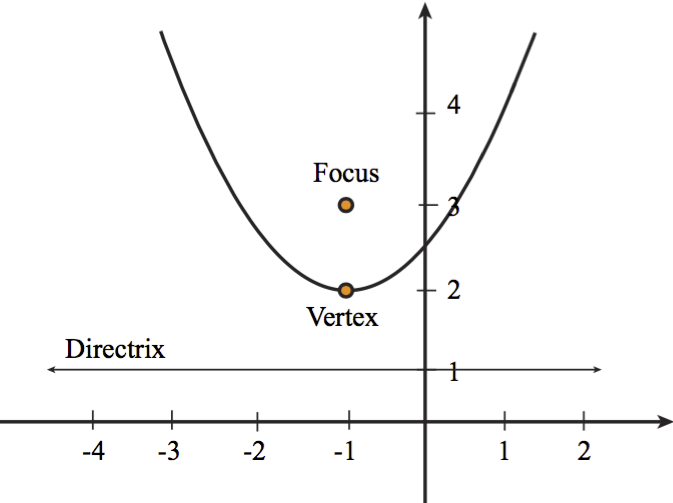
The equation for this parabola is 4(y – 2) = (x + 1)2. That equation is a little funny looking, although it isn't really polite to say that. We suggest you apologize. Anyway, it's because the equation is actually in the conic form for a parabola. That's the form:
4p(y – k) = (x – h)2
We recognize h and k from the vertex form of a parabola as, well, the vertex, (h, k). They've kept that job, despite the company restructuring. The 4p stuck on the y term is a little trickier to figure out. Looking at the original equation, though, we see that p by itself equals 1.
Okay, here's what you do. Look at the graph. Look back at us. Now at the graph. See the focus? How far is it from the vertex? Now back at us. Stop looking at us, look at the directrix? How far is it from the vertex? Back at us. Back at the graph. Look at the monkey. Now back at us again.
p gives us the distance between the vertex and the focus and directrix. It's an equal distance from both. For this graph, p is positive, so the parabola opens up. If your p is negative, though, things go south quickly. Strange accidents, bad luck, and parabolas pointing down are a few signs of negative p. See a licensed mathematician if you suspect your p has gone negative.
Sample Problem
Find the vertex, p, focus, and directrix of -8(x – 2) = y2.
Okay, this is a little disorienting. Having y be squared, instead of x? Weird, weird, weird. Well, whatever. It just means that we have a sideways parabola instead. The only major changes are that y is being squared, not x, and the 4p is attached to x now.
Our parabola will open to either the left or to the right. For our normal parabolas, a positive p opens in the direction of positive y (up). We do the same kind of thing here: a positive p opens in the direction of positive x, to the right.
Well, not here here. We have a negative p, so the parabola will open to the left. Not to the left of Albuqurque, this time, but from the vertex, at (h, k). We still have h with x and k with y, so the vertex is at (2, 0).
Next up is the value of p. The -8 attached to x equals 4p. So, solving 4p = -8 nets us a p = -2. This is supposed to be a distance, though, and we never have negative distances. We've never run a negative mile, and we doubt you have either. So we'll use its absolute value, 2.
Let's focus on the focus first. It is 2 away from the vertex, but is it 2 up, down, left, or right? It's a good thing those are our only choices, or we would really be in a pickle. Probably a dill pickle, if past experience is anything to go by.
The focus is always inside the arms of the parabola, and ours is opening to the left. So, the focus must be to the left of the vertex. It is 2 to the left, or -2 on the x-axis. Starting from the vertex, we go (2 – 2, 0) = (0, 0). The focus is focused on the origin.
We're not out of the brine yet. We still need the directrix, but it is very easy to find now. We start from the vertex and walk in the opposite direction from the focus. It's to the left, so we hike right 2. Remember that it's a line, though, so the directrix is x = 4.
We now have everything we need to graph this parabola. To fill it in, just remember that we start at the vertex, point away from the directrix, and curve around the focus. We'd suggest adding the line of symmetry through all of them, too, to keep it on track, but it happens to be the x-axis. There's no avoiding drawing it this time.

There we go, every piece in place just like we said. If you really want to be a stickler for accuracy, either plot another point or two or use the fact that the parabola has to be an equal distance from the focus and the directrix.
Swing Your Parabola, From Side to Side
Here are a few tips for working with parabolas in conic form. Step 1 is admitting we have a problem. Now here's the rest of what we should do.
- Find the vertex first. The x-coordinate is always by x, and the y-coordinate is always by y. Just be sure to get the sign right, or who knows where you'll end up. Maybe *gasp* Canada?
- The squared part of the equation determines if the parabola opens up/down or right/left. If we have y = x2, then it's the stand-up parabolas we know and love. If it's x = y2, shift your perspective to work with a sideways parabola.
- Finding p gives us the distance between the vertex and the focus and the vertex and the directrix. It's a twofer. The value 4p is attached to the unsquared part of the equation, so divide that by 4 to get to p.
- A positive p points up or right, while a negative p points down or left.
- The focus is always inside the parabola. Always. So if the parabola opens up, the focus will be even higher. If the parabola opens left, go even left-er to find the focus.
- Once you've found the focus, turn right back around to find the directrix. It is p away from the vertex in the opposite direction.
One last thing we might need to do is go from the quadratic form of a parabola to the conic form. We can't make this conversion at a bank, we have to rummage into that attic we call a brain and pull out a technique called completing the square. If you can't find it, check behind the stack of old records in the corner.
Sample Problem
Convert x = 2y2 – 8y + 24 into conic form.
It's been a while since we've messed with a quadratic equation. We're feeling either nostalgia or gas, not sure which. Anyway, to get this into conic form, we need to gather up our y and y2 terms into one big, squared term. That's where completing the square comes in. Even if the equation doesn't start off factorable, completing the square still gets us there in the end.
We'll start off by factoring the 2 out of everything on the right-hand side. We can't complete the square unless our squared term has a 1 as its coefficient.
x = 2(y2 – 4y + 12)
Now we find  . This is what we add to our y terms, and subtract from everything else, to create our square. It may look like magic, pulling numbers from nowhere, but it's actually addition by 0.
. This is what we add to our y terms, and subtract from everything else, to create our square. It may look like magic, pulling numbers from nowhere, but it's actually addition by 0.
x = 2(y2 – 4y + (4 – 4) + 12)
x = 2((y2 – 4y + 4) + (-4 + 12))
x = 2((y – 2)2 + 8)
Completing the square doesn't solve the problem, it just gets us close. We're so close, we can smell the answer, which is kind of gross, and also doesn't count in math class. To finish it off, we need to collect all of the constants on the same side as x.
½x – 8 = (y – 2)2
½(x – 16) = (y – 2)2
There we go. Now it's solved, and we don't have to smell it anymore. We have one piece of parting advice for this kind of problem. If we compare the first term of the quadratic equation, a, with 4p in the conic equation, we see that:

We can use this to check our results. If this equation isn't true for you, then you must have made a mistake, probably while completing the square.