Continuity - At A Glance

They're smooth as a baby's bottom, and not nearly as prone to smelly accidents. That's why we prefer continuous functions. We've thrown that term around a few times already, without really discussing what it means.

A continuous function has no breaks or points of discontinuity. They can be drawn without lifting our pencil, or our finger if we're drawing on an iPad. There are three main ways that functions can interrupt our doodling.

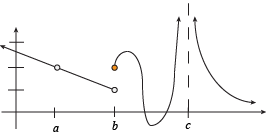
The first way, at x = a, is a hole. The hole can't be filled in, so we have to pick up and go around it. These are sometimes called point discontinuities. Second, at x = b, there is a jump discontinuity. The function literally jumps from one point to another. The third way is the vertical asymptote at x = c. Like some vampires and running water, functions refuse to cross vertical asymptotes.

Sample Problem

Locate and classify the points of discontinuity for .

We already know how to find every kind of discontinuity. We use the same techniques for finding holes and vertical asymptotes as we always have: check the denominators of fractions for zeros. Jump discontinuities are found when a piecewise function doesn't fit together properly, like a jigsaw puzzle that a 6-month-old has chewed on.

Starting on the first part of the function, 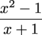, plugging in x = -1 leads to a zero in the denominator. We can factor the top into (x + 1)(x – 1), which cancels it out, though, so we have a hole, not a vertical asymptote. Just be careful not to fall in.

We have nothing to fear from 2x + 1, except fear itself, so we'll turn to x = 0. The function as we approach 0 from the left is going to be -1, while from the right it is 1. So this is a jump discontinuity.

The next point of contention is going to be at x = 0. The limit as we approach 0 from the left is going to be -1 while the limit as we approach 0 from the right is 1. So we're going to have a discontinuity. There definitely isn't a hole here and no asymptote either. This means this must be a jump discontinuity, too.

The Formal Definition of a Baby's Butt

Now that we know some more about discontinuity, we can bring things back to continuity—and limits. One of the major applications of all this limit business is to help show whether a function, at a particular point, is baby smooth or out of whack.

We have a function, f(x), and some point, x = c. To show that f(x) is continuous at c, we need to show three things:

1) 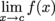exists

2) f(c) exists

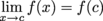
Or to put it in words:

1) The limit as x approaches c exists.

2) The function exists at c.

3) The limit equals the function at x = c.

In truth, we only need to show #3, because it includes the other two requirements inside it. We'll do an example going through all the steps in painstaking detail, though, just to be sure it all makes sense.

Sample Problem

Show that f(x) = x² is continuous at x = 2 using the formal definition of continuity.

We have three hoops to jump through. If the function makes it through all three, then the function is continuous, and the ringmaster will give us a salmon. Yum.

First, does the limit exist at x = 2? We can use direct substitution to show that it does, and the limit equals 4. Sometimes we'll have to check both the one-sided limits and see if they agree, but not this time.

Second, does f(2) exist? Totally, and it equals 4 as well. The fact that we used direct substitution to find the limit kind of gave that away.

Because the first two pieces were true and both equal to 4, the third piece of the continuity puzzle follows pretty naturally. This isn't surprising, because x² is a fairly well-behaved function. Good function; we'll share our salmon with you.

Let's look at a not-so-nice graph instead?

What if we had to check the continuity of this piecewise function at x = 1? Going from the left and right, we can see that the limit exists and equals 3. That's good.

Plus, the function itself exists at (1, 1.5). That's even better.

Then the function has an existential crisis, because the limit and the function don't agree on what happens at x = 1. The function is not continuous there, and it might want to see a therapist.

Sample Problem

Determine if 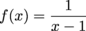is continuous at x = 1 using the formal definition of continuity.

Coming from the left of x = 1, the limit of (x – 1) is negative and gets smaller and smaller the closer to 1 it becomes. It's in the denominator, so that's a recipe for -∞. Swooping in close from the right, though, (x – 1) becomes smaller and smaller, but it stays positive; the limit there is +∞. The two sides conflict, so the limit Does Not Exist at x = 1.

We don't even have to talk about the fact that the function doesn't exist at x = 1. This function is not continuous. The vertical asymptote sitting at x = 1 puts the final nail in continuity's coffin.

All of our examples here could have been caught by sleuthing around for holes, jumps, and vertical asymptotes, so why do we even bother with the formal definition of continuity? Mathematicians hate formal occasions, so it can't be that they like it.

There are other kinds of discontinuity, and this definition will catch those, too. We just want you to be prepared in case an unfamiliar discontinuity tries to jump you in an alleyway.