High School: Geometry
High School: Geometry
Similarity, Right Triangles, and Trigonometry HSG-SRT.B.4
4. Prove theorems about triangles. Theorems include: a line parallel to one side of a triangle divides the other two proportionally, and conversely; the Pythagorean Theorem proved using triangle similarity.
When Harry and Helen started out, they hung things on blank walls and picked out tiles together. But, like all couples who break up, they had to split up their stuff in a way that they felt was fair and proportional.
Proportional splitting seems to be a trend amongst triangles when they are cut apart. Oddly, it's a common thread in the theorems about said triangles as well—making sure the parts are distributed proportionally when they do get cut apart.
Whether it's the Side Splitter Theorem claiming that a line parallel to one side divides the other two sides proportionally, or the Angle Bisector Theorem insisting that the resultant side segment pieces are proportional when one angle is bisected, triangles care more than any shape we know about fairness.
Students should know that in triangles and in life, fair doesn't necessarily mean equal. The pieces of the triangle are proportional, but that doesn't always mean congruent. They each get the right amount according to their own proportional needs. (Does this make them Marxist?)
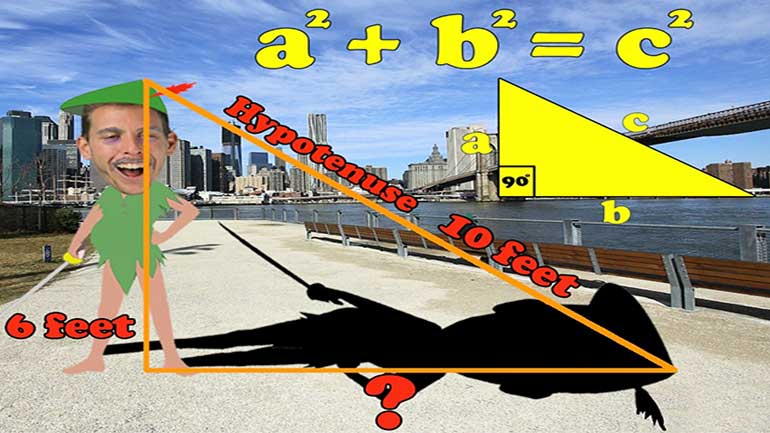
As for the Pythagorean theorem, well, that's not implicitly about splitting things up, but if we intend to prove it using similar triangles (which we do), then we will be explicitly splitting things up. We're talking about the fact that to prove similarity, we should look at ratios of side lengths rather than the lengths themselves.
Students will probably want to keep in mind the importance of being similar. Not to each other—though they will certainly be on top of the mismatched socks trend anyway—but the similarity between triangles that are, by definition, similar.
If they forget the basics of triangle similarity, give them a quick review of corresponding angles, corresponding sides, congruence transformations, and similarity transformations. These are pretty much the fundamental blocks with which students can build proofs about triangles.
Furthermore, students should definitely be able to name the three tests for identifying similar triangles: AA, SSS, and SAS. Remind them that when we're talking about similarity, those S's are more about the right proportions than they are about equality. Just like Harry and Helen.